Besides he photoelectric effect, usually the next objection to the idea that light itself is not intrinsically quantized (but that it is due to emission/absorption processes) is the “blackbody” spectrum, in particular, the “ultraviolet catastrophy”.

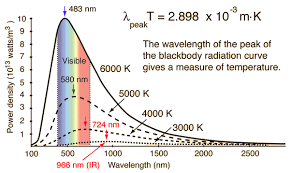
The “Ultraviolet Catastrophe”

The “ultraviolet catastrophe” is a term that was used to describe a problem in physics that arose at the end of the 19th century and the beginning of the 20th century. It refers to the prediction made by classical physics that a “black body” (an idealized physical body that absorbs all incident electromagnetic radiation) would emit radiation with infinite power. This prediction was in stark contrast to experimental observations, which showed that the power emitted at high frequencies (such as ultraviolet light) was much lower than predicted.

The term “catastrophe” was used because this prediction was a serious problem for the physics of the time. It indicated that there was a fundamental flaw in the understanding of energy distribution among the modes of a vibrating system, such as a black body. (Obvoiusly, no system can really have infinite energies, it makes no sense no matter how you look at it…). It really came about because the model used was way too simple, it was based on extremely simple idea that we just count the number of “modes” of a wave in a box…

The problem was resolved by Max Planck in 1900 when he introduced the concept of “quantization” of energy. Planck proposed that energy is emitted or absorbed in discrete packets or “quanta,” rather than continuously. This idea, which was a significant departure from classical physics, led to the development of quantum mechanics. Planck’s law of black body radiation, which includes this concept of quantization, accurately describes the observed black body spectrum, including the decrease in power at high frequencies, thus resolving the ultraviolet catastrophe.

Note: at this time, people did not know yet that electron energy levels were quantized! It was only later, with the work of Bohr and others, that the physical basis for this quantization was understood in terms of the behavior of electrons in atoms.

Electron Energy Levels

To understand this, we need to first think about what it means, why a warm body would emit any light in the first place.

When you heat an object, you’re essentially providing it with energy. This energy is transferred to the atoms that make up the object. The atoms begin to vibrate more energetically because of this additional energy.

Electron Excitation: The added energy causes the electrons in the atoms to jump to higher energy levels. These levels are often termed as “excited” states because they contain more energy than the electron’s original or “ground” state.

Relaxation and Photon Emission: The excited electrons don’t stay in the higher energy levels for long. They spontaneously drop back down to their original energy level, or sometimes to an intermediate level. The energy difference between these levels is emitted as a “photon” (note that these energy levels are of ocurse quantized). The energy of this “photon” (and therefore its color) corresponds to the energy difference between the two levels.

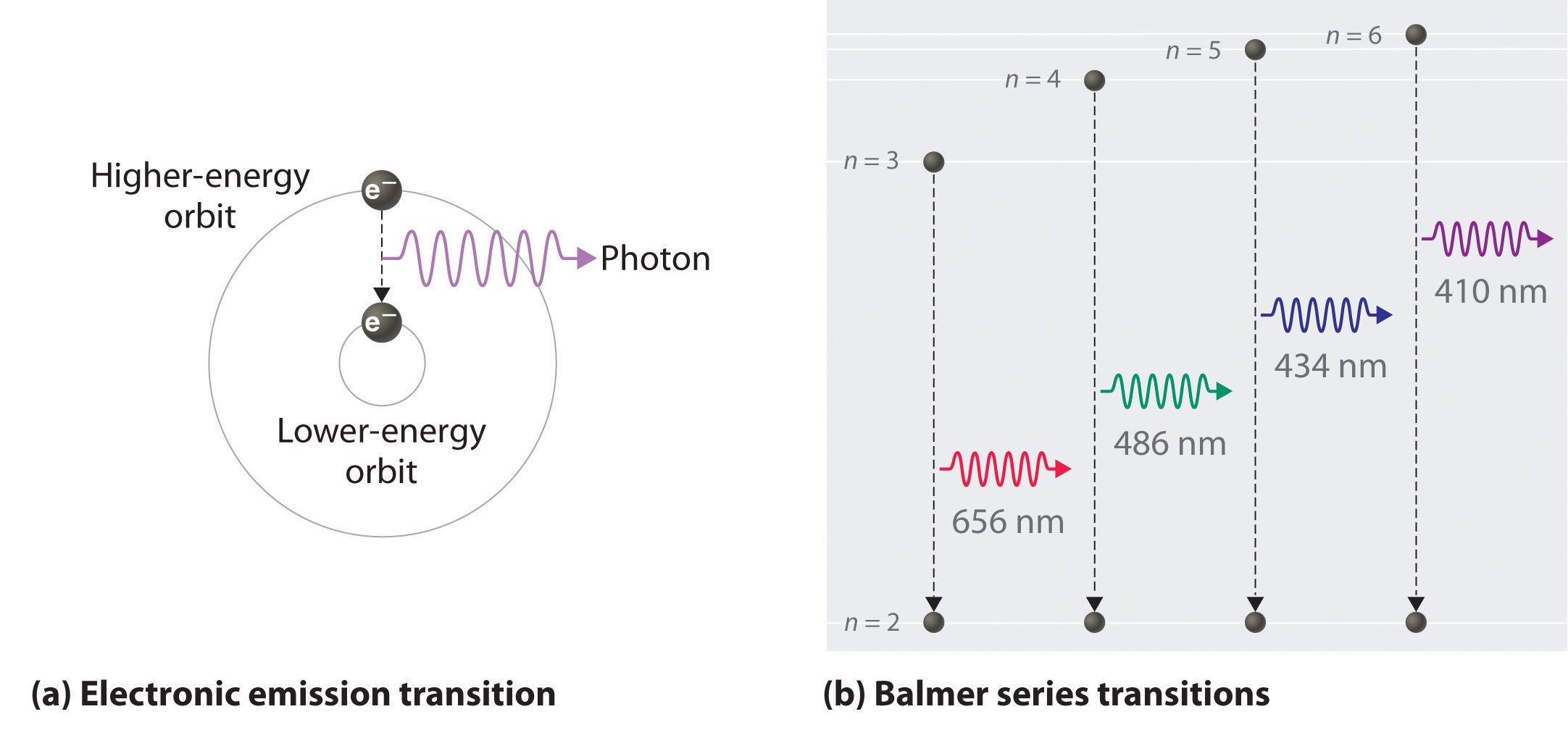
Visible Light: If the energy difference falls within the visible part of the electromagnetic spectrum, we see this as light. If the energy is lower (like in a warm object), it might be in the infrared range and we perceive it as heat. If the energy is much higher (like in very hot stars), it might fall in the ultraviolet range.

Thus, when you heat something up, like a piece of metal, it first starts to glow red (lower energy), then orange, and then white as it gets hotter and hotter. This is because it’s emitting more photons in the visible range, and across a wider spectrum of colors, as it heats up.

This is also the basis for the field of “thermography” where infrared cameras are used to detect heat differences. The hotter an object, the more it radiates energy, and not just in the visible part of the spectrum.

So, in essence, the process of heat turning into light is due to the behavior of electrons in atoms when they absorb and then release energy.

And that part is quantized!

Electrons exist in discrete energy levels, determined by solutions to the Schrödinger equation. These solutions are spherical harmonics ( among other mathematical forms).

The formulas can be found here: https://en.wikipedia.org/wiki/Table_of_spherical_harmonics

Here are the formulas for the first two l:

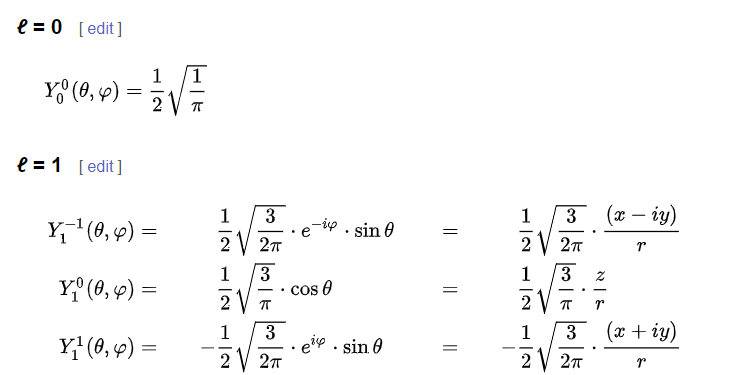
To excite an electron from one energy level to another, a specific amount of energy is required, corresponding to the energy difference between the two levels. Thus, only photons with the right frequency (and therefore the right energy) can cause an electron to transition between specific energy levels.

When an excited electron drops back down to a lower energy level, it releases energy in the form of a photon. The energy (and therefore the frequency) of this photon corresponds to the energy difference between the two levels .

Hence the quantization of energy levels embodied in the behavior of atomic electrons and their spherical harmonics, prevents the “ultraviolet catastrophe.” This is because energy cannot be emitted or absorbed continuously, but only in specific amounts corresponding to transitions between these discrete energy levels. This quantization of energy results in a finite, rather than infinite, amount of energy being emitted at high frequencies, such as in the ultraviolet range, thus averting the “catastrophe”.

Hence, the blackbody spectrum is perfectly compatible with a light that is not intrinsically quantized. The origin of the quantization are the discrete energy levels of electrons, which are based on the sperhical haromics solutions of Schrödingers equation.